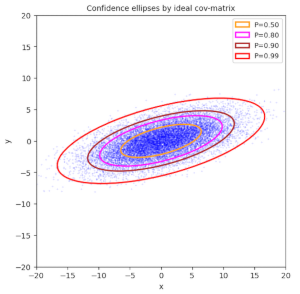
Compute confidence ellipses – II – equivalence of Schelp’s basic construction method for confidence ellipse with other approaches
In the 1st post of this series, I have motivated a simple method for constructing confidence ellipses for assumedly Bivariate Normal Distributions [BVD] or at least approximate BVDs. A reader has asked me, whether one can prove more rigidly that the proposed method of C. Schelp is equivalent to other BVD-based methods. Well, in this post we show that the… Read More »Compute confidence ellipses – II – equivalence of Schelp’s basic construction method for confidence ellipse with other approaches